11/13/19, rev 3/9/22,9/15/22,11/7/22,4/21/24,4/26/24,5/24/24,8/26/24,9/2/24,10/1/24

Water Vapor vs CO₂ for Planet Warming

Dan Pangburn, P.E. (ret), MSME, ASME life member

Abstract

During at least the time period when water vapor (WV) and carbon dioxide (CO₂) have been accurately measured worldwide, 1988-now, and apparently for centuries, WV increase has been responsible for the human contribution to Global Warming with no significant net contribution from CO₂ or any other greenhouse gases.

Introduction

A useful insight to the influence of WV on planet warming can be obtained from understanding why cloudless nights cool faster and farther when absolute water vapor content of the atmosphere is lower; especially when there is no dew or frost. This simple observation demonstrates that water vapor is infrared electromagnetic radiation (IR) active which makes it a so-called greenhouse gas (ghg AKA RAG for Radiantly Active Gas), thermalization takes place (ghg molecules absorbing radiant heat from the surface or other ghg and sharing the absorbed energy with surrounding molecules), and that the misleadingly named greenhouse effect (GHE) exists i.e. the planet surface is warmer with the presence of WV than it would be without it.

The words ‘water vapor’ can be a bit misleading. WV is a transparent gas. If something is visible, like steam or a cloud, it is not WV but is condensed liquid water droplets or tiny bits of ice.

The term ‘vapor pressure’ has different meanings in different disciplines. In meteorology it means the partial pressure of WV in the atmosphere. In most other disciplines and general use, it means the pressure developed by the liquid as a result of its impetus to change phase and become a gas. This impetus depends only on the temperature of the liquid water. It is unambiguously called saturation vapor pressure. The pressure of WV in the atmosphere is identified as its partial pressure.

Another difference in term usage is the meaning of the word ‘feedback’. In engineering it usually refers to feedback factor, a dimensionless number which is the ratio minus 1 of response with feedback to the response if there were no feedback. In most science disciplines it refers to the magnitude of the response to a forcing which might or might not influence the cause of the forcing. In Climate Science it is measured in W/m².

1. Atmospheric structure

At a scale of the size of atoms the atmosphere consists of spinning and vibrating gas molecules, with empty space between them, bouncing elastically off surfaces and each other. Activity of all of the gas molecules determines properties which can be measured such as temperature and pressure. Only certain gas molecules significantly contribute to radiation heat transfer. These are IR active in the wavelength range of earth temperatures, are misleadingly called greenhouse gasses (ghg) and include CO₂ and WV. The radiation energy travels from ghg molecule to ghg molecule (or between surface and ghg) at the speed of light (in the atmosphere which is 99.97 % of the speed of light in a vacuum) but effectively dwells in each CO₂ molecule for up to about 1.1 second making the molecule warmer. The increased cumulative dwell time from increased WV molecules is what causes the increased Greenhouse Effect (GHE). Dwell time is AKA decay time. 

Approximately 99% of dry atmospheric molecules are non-ghg; nearly all nitrogen and oxygen with about 1% argon. Near the surface, they are substantially warmed by thermalization of the earth-source thermal radiation energy absorbed by the ghg molecules and, at higher altitudes (starting a few meters or less above the surface), cooled by pressure decline and reverse-thermalization back to the ghg molecules. Above about 2 km altitude, because of steep decline of the population of WV molecules, lower pressure, and wider spacing between ghg molecules, outward directed radiation from WV molecules can make it all the way to space.

All molecule species are fairly well mixed throughout the atmosphere with the exception of WV. WV molecule population declines with altitude from average of about 8,000 ppmv (parts per million by volume) at sea level [34, 35] to, because of the low temperature (~ -50 °C, saturation vapor pressure of ice 3.94 Pa [28] and total pressure at 12 km of 19,400 Pa), to a maximum of about 3.94/19,400 = 0.000203 = 203 ppmv at the tropopause. The 8,000 ppmv (0.8%) average increases to about 4% in the tropics.

The tropopause altitude averages about 12 km (39,370 ft) with up to 16 km or so at the equator. In addition to the population decline of WV molecules due to temperature decline, is the decline due to average pressure decline of 19.4/101.3 = 0.19 from a sea level pressure of 101.3 kPa. The combination results in an overall average WV molecule population decline up to the tropopause of about 8,000/203 * 101.3/19.4 = 206 to 1.

 

2. Thermalization

Thermalization and/or reverse-thermalization (thermal conduction from surrounding molecules to replenish energy radiated by a ghg molecule) occur continuously throughout the atmosphere. Typical TOA emission spectra are shown in Figure 1. A black-body emission curve (emissivity 1) for an average global temperature of 288 K is a bit higher than a curve for the actual surface which has an emissivity about 0.99. The TOA flux is the black trace in Fig 1. The TOA and surface curves show radiation flux being slowed between the two locations (absorbed/emitted by all ghg). On average, up to about 1.1 sec passes between absorption and emission of a photon by a molecule. Prominently shown is the ‘notch’ associated with the CO₂ absorb/emit band. The actual surface curve minus the TOA curve results in what is called the Greenhouse Effect (GHE).

 A much more extensive description of thermalization is at Section 4 of [19].

Figure 1: Thermal radiation from below assessed from top-of-atmosphere. Lower wavenumber photons are lower energy. (Graph is from NASA [1])

3. Relative influence of CO₂ and WV on climate

Hitran [2] using Quantum Mechanics and measurements determines, besides many other things, the relative absorb/emit intensity of water vapor molecules vs CO₂ molecules. Comparison at zero altitude, when scaled by atmospheric abundance, is shown in Figure 2. At sea level, WV molecules outnumber CO₂ molecules by about 8,000/330 ≈ 24 to one. 

Figure 2: At zero altitude, CO₂ absorb/emit is barely discernable compared to WV.

The relative increase of WV and CO₂ over 30 years is calculated as follows:

CO₂ increase in 3 decades [3], 1988 to 2018: 407 - 348 = 59 ppmv

 Average global water vapor increase trend from Figure 5, which is a graph of NASA/RSS TPW data, is 0.0416/29 * 100 * 10 = 1.43 % per decade.

 The ground level population of WV molecules averaged about 8000 [34, 35]. Figure 3, at 30 degrees latitude (area to pole = area to equator) also averages global WV ≈ 8,000 ppmv. WV increase in 3 decades = 0.0143 * 8,000 * 3 = 343 ppmv.

Figure 3: Water vapor declines with latitude and rapidly with altitude. [4] (original from NASA)

Therefore, WV increase has been 343/59 ≈ 5.8 times more than CO₂ increase 1988-2018. (Much of the world human population has been falsely indoctrinated)

Above about 2 km and from below wavenumber 600, outward directed emission of WV can make it all the way to space. Some of the energy absorbed by CO₂ molecules below the tropopause is redirected with respect to wavenumber via thermalization to WV molecules. Schwarzschild’s equation [40] accounts for this by assuming that “as radiation passes through an isothermal layer, its monochromatic intensity exponentially approaches that of blackbody radiation corresponding to the temperature of the layer.”

Well above the tropopause, radiation emitted from molecules there to space is primarily from CO₂ molecules (partially indicated in Figure 1 by the spike at wavenumber 667). If you ignore the increase in water vapor (big mistake), near the surface, WV averages about 8,000 ppmv.  WV above the tropopause is limited to about 203 ppmv because of the low temperature (~ -50 °C) while the CO₂ fraction remains essentially constant with altitude at (in 2019) about 410 ppmv; up from about 295 ppmv in 1900. The increase in absorbers at ground level since 1900 is then about 8,410/8,295 ≈ 1%. The increase in emitters to space at high altitude (~> 20 km, 0.055 atm), and accounting for the lower atmospheric pressure, is (410 + 203)/(295 + 203) * 0.055 ≈ 0.068 = 6.8%. This (6.8%>1%) explains why CO₂ increase does not cause significant warming (except near the poles). The result being that Climate Sensitivity (the temperature increase resulting from doubling the CO₂ level) is not significantly different from zero.

 More evidence that CO₂ has no significant effect on climate is at Sect 2 of [19].

 The exception at the poles is because it’s cold there at ground level so WV molecule count is already low. Therefore, transfer of energy to WV molecules which radiate it to space is negligible.

4. Water vapor increase is a cause and also a result of warming

WV increase is a cause of warming (average global temperature increasing) because it is a ghg. Part of WV increase is a result of surface water warming because its saturation vapor pressure increases with temperature. The saturation vapor pressure increase causes an increase in the rate of WV molecules being forced into the atmosphere (when the atmosphere at ground level is less than saturated with WV which is usually the case). Additional sources of WV increase include human activity, especially irrigation due to increase in residence time, and decrease in condensing out WV in cold ocean waters. This is discussed further in Section 6.

 In the atmosphere, condensed water can exist as water, ice or super-cooled water [26] (super-cooled water is liquid water below 0.0 °C). Accurate numerical values for saturation vapor pressure of liquid water [27] and ice [28] are graphed in Figure 4. Saturation vapor pressure for super-cooled water can be calculated using the Bolton equation [25]. The Bolton equation for saturation vapor pressure in kPa vs temperature in C is

p = 0.6112 * e^(17.67 * T / (T+243.5))                      (1)

 As shown in Figure 4, saturation vapor pressure increases progressively with temperature. Of interest is the % increase in saturation vapor pressure per degree increase in temperature. This is readily calculated from the numerical data for both liquid water and ice from:

1/1 increase/Tave = (p_j – p_(j-1)/(T_j – T_(j-1))/Tave                        (2)

Where,

1/1 = %/100

j and (j – 1) are adjacent values in the table

Tave = average temperature of the adjacent values.

 

The same thing for super-cooled water is obtained using the first derivative of the Bolton equation which is

dp/dT = p * 17.67 * 243.5/(T+243.5)^2                     (3).

 

This, divided by p to get the 1/1 value curve, is shown in the bottom graph at Fig. 4.

 

Saturation vapor pressure depends ONLY on the temperature of the ice or liquid water. The 1/1 change in saturation vapor pressure per Celsius degree for water, ice and super-cooled water are shown in the lower graph of Figure 4. 

Figure 4: Saturation vapor pressure of ice &water and fractional rate of change per C degree change vs temperature.

The atmospheric temperature decreases with altitude so the accommodation for WV increases with altitude to about 12%/C° at the tropopause (°C is a temperature, C° is a temperature difference and is used interchangeably with K for Kelvin degree). Although the accommodation per degree increases with altitude, the magnitude of a temperature change usually decreases with altitude faster with the result that as absolute humidity increases, relative humidity usually slightly also increases.

Based on ocean temperatures from [29], the area-weighted change in saturation vapor pressure per C degree at sea level is about 0.0633 / C°. The amount of compounding is unknown but cannot be greater than 0.0633+0.0633^2+0.0633^3+… = 0.0676/C°. It is conservatively estimated to be about 0.067/C° = 6.7%/C°

5. Measured water vapor increase

The accelerated increase in WV, above that determined from just global temperature increase, is expected from the surge in irrigation and human population with accompanying increase in WV residence time and has also been measured. Average measured global atmospheric water vapor (total from surface to TOA) over the years is provided here at Figure 5.

 Clear sky water vapor measurements over the non-ice-covered oceans in the form of total precipitable water (TPW) have been made since Jan 1988 by Remote Sensing Systems (NASA/RSS) [5]. A graph of this measured ‘global’ average anomaly data, with a reference value of 28.73 added [24], is shown in the left graph of Figure 5. This data is extrapolated earlier using CO₂ level as a proxy, with the expression kg/m^2 TPW = 4.5118 * ppmvCO₂^0.31286. This matches the magnitude in Jan 1988 and slope of the trend of measured WV. The result is the right-hand graph of Figure 5 which shows approximately 6% increase 1960-2010. 

Figure 5: Average clear air Total Precipitable Water over all non-ice-covered oceans as measured by NASA/RSS using satellite based instrumentation and with extrapolation by me. (Left graph is by month, right graph is by year average.). Estimated near future minimum trend is 29.7 mm. Estimated near future maximum is the slope of the recent monthly trend.

Both WV and CO₂ have increased since before both have been accurately measured worldwide. The CO₂ is well mixed and its increase is directly reported in ppmv [3]. Water vapor increase is measured and reported in kg/m^2 [5]. Average WV is about 29 kg/m^2. The WV increase in ppmv is obtained from the TPW anomaly data by first calculating the fraction of the atmosphere that is WV. The weight of the atmosphere is 14.696 psi = 10332 kg/m^2 so the fraction by weight is 29/10332 = 0.002807. This times the ratio of molecular weights gives the average WV for the entire atmosphere in ppmv. WV = 28.96/18.015 * 0.002807 = 0.004512 or 4512 ppmv. The values for ppmv WV are then the values by weight (plus an offset of 0.48 kg/m^2 to get the ppmv to start from zero) times 4512 ppmv/29 kg/m^2. This is shown in Figure 5.1 along with the values for CO₂. The ratio of the slopes discloses that, in the total atmosphere, WV molecules have been increasing about 6.4739/2.0459 = 3.16 times faster than CO₂ molecules.

The same calculation at ground level, because of the higher level of WV (about 8000 ppmv), results in WV increasing there about 5.6 times faster than CO₂.

Figure 5.1: Comparison of WV increase in ppmv and CO2 increase in ppmv in the total atmosphere.

6. World Sources of Increased Water Vapor

Irrigation, industrialization, and, increasing population have been contributing (later found to be insignificant) to the rise in atmospheric WV above that due to liquid water temperature increase. A survey of available on-line literature provides direct and indirect quantification of significant global sources of the extra increase.

Transportation fuel, linearly interpolated to 2017, amounts to 113E15 BTU/y [6]. Energy content of a typical liquid fuel is 115,000 BTU/gal [7]. Liquid fuels weigh about 6.073 lb/gal = 2.75 kg/gal. Therefore transportation fuels amount to

113E15 * 2.75/115000 = 2.7E12 kg fuel/y                 (a)

About 1.42 kg of WV is produced for each kg of liquid fuel [7] so the amount of WV produced by transportation is

2.7E12 * 1.42 = 3.8E12 kg WV/y                  (b)

World electricity generation is now about 25,000 TWH/y [8]. At an average efficiency of 50% and ignoring non-thermal sources this requires a thermal input of 50,000 TWH/yr. Fuel source fractions of energy [9] in 2017 are approximately 0.38 coal, 0.36 natural gas and 0.26 non fossil fuel.

Coal combustion produces about 0.4 kg WV/kg coal [10]. Energy content of bituminous coal is about 8200Wh/kg [11]. The amount of WV resulting from burning coal to generate electricity is then

50E15 * 0.38 * 0.4/8200 = 0.93E12 kg WV/y                       (c)

The amount of WV produced by natural gas (nearly all methane, CH₄) is readily calculated from the dominant chemical reaction

CH₄ + 2O₂ => CO₂ + 2H₂O                (d)

Where a mole of methane weighs about 16 g and the two moles of WV weigh about 18 g each.

Natural gas energy content is about 15,400 Wh/kg [11]. The amount of WV resulting from burning natural gas to generate electricity is then

50E15 * 0.36 *36/16/15400 = 2.6E12 kg WV/y                    (e)

The total WV from all fossil fuel used to generate electricity is then

0.9E12 + 2.6E12 = 3.5E12 kg WV/y                         (f)

Waste energy during electricity generation can be approximately accounted for by evaporation of water in cooling towers, etc. At 50% efficiency the waste energy is the same as the energy in the electricity produced, 25,000 TWH/yr = 25E12 kWh/y.

Latent heat of water = 2257 kJ/kg = 0.627 kWh/kg = 1.594 kg/kWh.

The amount of WV from waste heat (cooling tower, etc.) during electricity generation is then

25E12 * 1.594 = 39.8E12 kg WV/y                           (g)

Irrigation is by far the largest source of WV. The increase in irrigation is indicated by the increase in withdrawal for agriculture as shown in Figure 6 [12].

Figure 6: Global water withdrawal includes both ground water and surface water [12]

The total agricultural area equipped for irrigation in 2012 was 324E10 m² [13]. Estimate 80% were actually being irrigated. Estimating an increase of 2% to 2017, the total area being irrigated is now about

324E10 * 0.8 * 1.02 = 266E10 m²                              (h)

This is more than 4 times the area of France and is probably warmer than the tropics.

Total annual fresh water withdrawal (both ground and surface) is now 3,986 km³ = 3.986E15 kg/y [14]. Of this, about 70% is for agricultural use [15]. This works out to

3.986E15 * 0.7/266E10 = 1052 kg/m²/y ≈ 1 m/y                   (i)

which appears reasonable because average rainfall for the planet is about 1 m/y.

Evapotranspiration, WV from plants and landscape, is discussed in the ‘thematic discussion’ of Aquastat [12]. From there, the amount of precipitation on land is 110,000 km³ /y of which the fraction evapotranspirated is 0.56 + 0.05 = 0.61. Given the planet surface area of 510.1E6 km², and land fraction of 0.29 this results in the equivalent liquid depth of the total amount of water leaving the surface as WV as

110,000 * 0.61/0.29/510.1E6 = 0.00045 km/y = 0.45 m/y                    (j)

Water weighs 1000 kg/m³ so evapotranspiration amounts to 450 kg/m² /y.

Worldwide about 86% of irrigated area is flood irrigated [20]. To simplify calculation, assume all irrigation is flood irrigation approximated as furrow type [16]. Optimum frequency is to flood the furrows about every 10 days [17]. Thus about half the area is covered by water 10% of the time where evaporation from the water is about one meter per year [18] and the rest of the time, the additional evaporation is assumed to be according to the calculated evapotranspiration. Evapotranspiration prior to irrigation must have been low or irrigation would not be done. Evapotranspiration with irrigation, to be cost effective, is most likely to be much more than calculated. These two uncertainties are assumed to approximately cancel each other. A further assumption is that, on average, irrigation is applied for about 1/3 year. The total amount of WV resulting from irrigation is then

[(0.1 * (1 + 0.45)/2 + 0.9 * 0.45) * 266E10]/3 = 42.3E10 m³ /y = 42.3E13 kg/y           (k)

 

These calculations are summarized in Table 1

 

Water vapor source	E13 kg/y	%
Irrigation	42.3	90.0 %
Transportation fuel	0.4	0.8 %
Fossil fuel for electricity generation	0.3	0.7 %
Cooling towers, etc. for electricity generation	4.0	8.5%
Total	47.0	100 %

Table 1: Summary of contributions to atmospheric water vapor.

WV increase above that due to feedback from liquid water temperature increase results about 90 % from irrigation. WV added by irrigation is particularly influential because it is added at locations where natural WV is low and because the liquid water is shallow, it is quickly and substantially warmed by the sun.

 The assessment here that irrigation accounts for 90% of humanity’s contribution to WV corroborates what Shiklomanov 1997 determined as mentioned by Doll in 2002 [33].

 The amount of WV put into the atmosphere by human activity is 47E13 kg/yr. On the planet with area 51E13 m^2 this is 47/51 = 0.92kg/m^2/yr = 0.92 mm/yr. Average annual rainfall is about a meter (1000 mm) so human activity only adds about 0.92/1000 = 0.00092 or about 0.09% to rainfall. (This was also calculated at the last paragraph of Section 9 in [19])

The oceans, especially the warm tropical areas, produced the WV responsible for the basic GHE temperature increase of 33 K. Figure 6.1 shows typical WV distribution over the planet.

Given that human activity is not contributing significantly to precipitation increase, the increase in WV must be due to increase in residence time of the WV. Average global precipitation in a year is about 1000 mm so the average residence time of WV molecules in the atmosphere in Jan 1988 was about (Fig 5) 28.2/1000*365 = 10.293 days. To get to 29.7 mm in Dec 2023 the residence time for the same rainfall is 29.7/1000*365 = 10.841 days for an increase of only 0.548 day in 36 years. The slightly longer residence time probably results because the WV is added at locations with low precipitation. This slight increase in residence time is reduced by whatever increase in WV is accounted for by increased temperature. The fraction of WV residence time increase accounted for by increased temperature varies from about 39% to 75% depending on which agency’s AGT is used..

 At about 35 degrees latitude from the equator the vapor pressure in the oceans is about the same as the average partial pressure of WV in the atmosphere. Beyond that, WV is sucked out of the atmosphere by cold ocean water. Warming of this cold ocean water would reduce the rate of absorption/condensation resulting in increase in WV. 

The lower panel in Figure 4 shows that the fractional rate of change of saturated vapor pressure is larger at lower temperature so the reduction of absorption per degree change in temperature is greater than the increase in evaporation in warm waters.

 

Figure 6.1: Typical WV distribution over the planet. [39]

As shown in Section 19 at [19], the measured increased WV, which includes humanity’s tiny contribution, has contributed an additional approximately 0.9 K of the total average global temperature increase since 1895. Ref [19] identifies the three contributing factors considered, the data sources, and the algorithm which calculates temperatures which match measured average global temperatures about 93% 1895-now (thru 2023).

7. Comparison of measured WV with WV increase calculated from feedback over a long time period.

Some people have asserted that WV content increases with air temperature. That would be true if the air was all continuously at saturation. Of course it is not. The driving factor for WV increase is the saturation vapor pressure increase due to temperature increase of the liquid water source. Increase of air temperature does add accommodation for the added WV. A NET driving factor in comparatively warm water within about 35 degrees latitude of the equator is the vapor pressure deficit which is the difference between the saturation vapor pressure of the liquid surface water and the partial pressure of WV in the atmosphere.

 As described in Section 4, as the temperature of liquid surface water increases, its saturation vapor pressure increases which, if the atmosphere is not already saturated, forces more WV molecules into the atmosphere. This contributes to a net feedback from all factors which caused the temperature to increase. A conservative value for WV increase (actual WV increase from feedback will be less than so calculated) was estimated in Sect 4 from available measured data to be 6.7% = 0.067 1/1. The large effective thermal capacitance of the planet is the main contributor to the conservatism. The temperature rise is in response to the time-integral of the forcing so it will lag the feedback forcing. 

The file for calculated change in WV due to temperature increase is generated in EXCEL where each row contains:

 WV_n = WV_(n-1) + (T_n – T_(n-1))* R * (WV_(n-1) + F)

 Where:

WV_n = calculated WV in month n, kg/m^2

T_n = temperature anomaly in month n, C°

R = effective rate of WV increase resulting from feedback of temperature increase, 0.067/C° (= 6.7 %/C°)

F = added to avoid circular reference of (WV_(n-1)+WV_n)/2. F is calculated as an increase to each month equal to half a month at the final slope. This requires iteration.

For HadCRUT5 [31] as of Dec 2021, F = 0.029835/24 = 0.00124 kg/m^2/month. Slope at F = 0 is 0.0298342. Effect over 34 yr = (0.0298355 - 0.0298342)*34 = 0.000044 kg/m^2

The starting calculated WV is adjusted to make the starting points of the trends the same.

The results of this algorithm are shown in Figure 7 along with the actual measured WV anomaly measured and reported by NASA/RSS [5] (plus 28.73). The measured WV is about 40.4% steeper than the calculated trend using HadCRUT5, Jan 1988 – Dec. 2023 temperature trend and 6.7%/K.

     

            0.4161/0.3105 = 1.34

Figure 7: Measured WV and calculated WV based on HadCRUT5 reported average global temperatures and compounded feedback.

The GCMs calculate the WV within the models with the result that relative humidity is approximately constant. The linear trends assuming three different values for average relative humidity remaining constant with increasing air temperature are included on Figure 7.1.

Figure 7.1: Same as Fig. 7 but with trends based on constant relative humidity added.

The observation that the actual measured trend is steeper than calculated trends demonstrates that, on the long term, measured WV is increasing faster than possible from just global average temperature increase of the liquid water (feedback). The steepest slope calculated for the constant RH cases is (29.36 – 28.242)/34 = 0.0329 kg/m^2/y. The measured trend is then 0.0416/0.0329 = 1.264 or about 26.4% steeper than the estimated trend calculated within GCMs. 

There must be other factor(s) contributing to WV increase. This is most likely due mostly to a slight increase in residence time as calculated in Sect 6. As introduced in Section 6, a contributing factor is reduction of the rate of absorption/condensation of WV into cold water. An even more basic consideration is that determining the influence of increased WV from just feedback from temperature increase is too low. The only valid consideration for the influence of WV increase is to use the measured WV increase or equivalent increase in residence time.

Except for UAH, reported temperatures by the several agencies are subject to uncertainties in estimated Heat Island Effect. UAH 6.1 temperature data are used in the calculation of atmospheric WV using the above algorithm. Results are shown in Fig 7.2 along with the measured WV. The difference in rates is the ratio of the slopes: 0.04161/0.01637=2.54. That is, the measured WV increase is about 150 % higher than possible from just planet warming.

Figure 7.2: Measured WV and calculated WV based on UAH 6.1 global temperatures [42]. (WV increase 6.7%/K)

 

The average increase in residence time was calculated at the end of each of several years using the UAH 6.1 temperature data. It settled down with time. The results are graphed in Figure 7.3. This shows that the average year-to-year increase in residence time is about 13 minutes. This accounts for the increasing trend of measured WV. The calculated results 2015 thru 2023 are: mean 12.9 minutes, S.D. 0.25 minutes.

Figure 7.3: Year-to-year increase in residence time

A corroboration of the long-term temperature trend is as follows: Assume that, at the beginning of the warm up, the temperature increase was caused by something else. Then the WV increase can be calculated from that temperature increase using the saturation vapor pressure vs temperature for water and the assumption that % increase in WV = % increase in saturation vapor pressure. But the WV has increased more than that so there has to be an additional cause of WV increase or a reduction of the condensation and/or absorption of WV by cold ocean water.

8. Over a short time period, water temperature drives WV.

Surface water temperature fluctuates as shown in an animation [21]. A particularly dominant fluctuation is in the equatorial Pacific and is tracked and reported weekly as el Nino. Fluctuations in el Nino affect short term global average WV and average global temperature as shown in Figure 8.

Figure 8: On the short term, local water temperature fluctuations drive global WV and average global temperature.

9. Influence of WV increase on HadCRUT5 average global temperature.

Figure 9 is a plot of the measured WV [5] vs measured HadCRUT5 data [31]. It shows the short term scatter as well as the long term trend of the influence of WV on average global temperature. The closeness of the yearly trends demonstrates that the data scatter is essentially symmetrical.

Figure 9: Scatter graph of WV vs HadCRUT5 measured data.

 

This provides fertile ground for those motivated to mislead to cherry-pick periods where the increasing side of fluctuations drives both WV and outgoing-longwave-radiation up.

10. Energy Redirection

Figure 10 shows TOA radiation flux intensity vs wavenumber as calculated by Modtran [22]. This calculated radiation flux profile has been corroborated by satellite measurements [23]. Superimposed on the graph are plots of black body radiation flux at specific temperatures. Black body radiation imposes an upper limit on radiation intensity at characteristic wavenumbers of each ghg.

Figure 10: Typical TOA radiant emission

Standard atmosphere tables show temperature vs altitude so the bb radiation curves are also altitude curves. The curves are very nearly equally spaced (the lapse rate) up to the tropopause. From this it is seen for example that all radiation emitted in the range 500-600/cm is from between the altitudes 2 – 6 km and that the outward directed radiation in this range can make it all the way to space.

Essentially all of this radiation (500 to 600/cm, 2 to 6 km) comes from WV molecules. The result is part of the energy absorbed by CO₂ molecules at this altitude range is shared with surrounding molecules via gaseous conduction and the fraction radiated outward by WV molecules can make it all the way to space. Effectively part of the radiation energy absorbed by CO₂ is redirected with respect to wavenumber to replenish energy radiated to space by WV. This process applies to all radiation absorbed by CO₂ molecules up to the tropopause.

11. Climate change without CO₂ change and CO₂ change without otherwise accounted for climate change.

A main concern from burning fossil fuels, especially coal, is the production of CO₂ which many believe is a primary contributor to climate change. Proxy measurements covering the Phanerozoic eon (last 540 million years) show substantial changes in CO₂ with no consistent correlation between CO₂ level and average global temperature, Fig 10.9.

Figure 10.9: CO₂ and average global temperature over geologic time (time scale is logarithmic). 

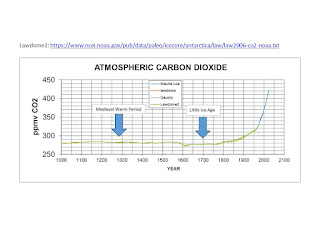
The atmospheric CO₂ level has been measured in ice cores extracted at Lawdome Antarctica [41]. Figure 11 shows the CO2 level at Lawdome since the year 1000. This shows that there was negligible CO₂ change in spite of the temperature fluctuation from the high of the MWP to the low of the LIA. Perhaps more pointedly, the planet experienced both the MWP and the LIA at very nearly the same CO₂ level of 280 ppmv.

Figure 11: Climate change from MWP high temperature thru LIA low temperature had little effect on CO₂ level,

In Figure 12, the temperature and CO₂ chart constructed from proxy data for the last 800,000 years and put together by NOAA, clearly shows several periods when temperature changes preceded CO₂ changes. During this period of comparatively low CO₂ level, the atmospheric CO2 level appears to have been determined by solubility of CO₂ in water.

 The chart to the right on Figure 12 is to the same vertical scale but expanded in time to show measured data since 1850. This shows a CO₂ increase of about 160 ppmv and rising, while temperature, although rising slightly, remains in the range of previous interglacials. The temperature increase of about 1.2 C is mostly explained by the increase in WV [19].

     

Figure 12: Current CO2 and temperature to paleo scales.

12. Summary

Section 17 of the analysis at [19] shows that about 2/3 of the average global temperature increase since 1909 can be attributed to the increase in Total Precipitable Water (TPW) (AKA Total Column Water). The increase in TPW is accurately measured by NASA/RSS using satellite-based instrumentation. They document TPW anomalies monthly in annual reports available on line. The latest report is available at [5]. The measured rate of increase trend of TPW through Dec 2023 is 0.0416 kg/m^2/year which is the slope of the regression line in Figure 5.

From Table 1 the amount of WV put into the atmosphere by human activity is about 47E13 kg/yr. On the planet with area 51E13 m^2 this is 47/51 = 0.92kg/m^2/yr = 0.92 mm/yr. Average annual rainfall is about a meter (1000 mm) so human activity only adds about 0.92/1000 = 0.00092 or about 0.09% to rainfall. (This was previously calculated at the last paragraph of Section 9 in [19]) Human activity is not contributing significantly to precipitation increase so the increased TPW must be due mostly to increase in residence time.

 The expected amount of WV increase from warm tropical water resulting from feedback from planet warming can be calculated from measured Average Global Temperature (AGT) (AKA Global Average Temperature or GAT and total column water) and the saturated vapor pressure vs temperature curve for water (Several analytic expressions have been derived over the years, always starting with the Clausius-Clapeyron relation, to approximate the saturation vapor pressure curve.) The graph at Figure 4 is very accurate.

One of the highest AGT assessments (if not THE highest) is reported as HadCRUT5. The WV increase from feedback that HadCRUT5 temperatures would produce is shown at Figure 7. The regression slope for this is 0.031 kg/m^2/yr. This indicates that other factor(s) contributing to WV increase must account for about 0.0416 – 0.031 = 0.01 kg/m^2/yr or an increase in residence time of about 0.01/29*10.5 day = 0.0036 day, about 5 minutes/year, 3 hours in 36 years.

A possible other factor is the reduction in absorption/condensation as a result of warming of cold water. In the summer at about 35 deg latitude from the equator the saturated vapor pressure in the ocean is about the same as the typical partial pressure of WV in the atmosphere. Colder water farther from the equator, instead of being a source for WV, is a sink. The cold ocean water condenses WV out of the atmosphere. Warming of this water would reduce the amount of WV drawn from the atmosphere contributing to this increase in WV. As shown in Fig 4, the warming of cold water increases WV about 7%/C deg compared to warm water at about 6%/C deg of temperature increase.

A low AGT increase assessment is by UAH. The WV increase from feedback that UAH temperatures would produce is shown at Figure 2.9 in the analysis at [19]. The regression slope for this is 0.0182 kg/m^2/yr. This indicates that the contribution from other causes must be about 0.0416 – 0.0182 = 0.023 kgm^2/yr or an increase in residence time of about 12 minutes each year compared to the previous year. The total increase in residence time in 36 years is about 12*36 = 432 minutes = 7.2 hr.

The total contribution to AGT increase 1909 thru 2023 from WV increase is about 2/3 * 1.35 = 0.9 K.

 Conclusion

Humanity’s contribution to precipitation and therefore WV increase is shown to be negligible so increased atmospheric water vapor is essentially all from increased AGT and increased residence time of the WV in the atmosphere. Increased CO₂ has negligible effect on warming. Climate Sensitivity, the temperature increase from doubling CO₂, is not significantly different from zero.

References:

1. NASA/GISS TOA graph source https://www.giss.nasa.gov/research/briefs/2010_schmidt_05/ 

2. HITRAN data base calculator http://www.spectralcalc.com/spectral_browser/db_intensity.php

3. Mauna Loa CO₂: ftp://aftp.cmdl.noaa.gov/products/trends/co2/co2_mm_mlo.txt

4. Water vapor vs altitude http://homeclimateanalysis.blogspot.com/2010/01/earth-radiator.html

5. NASA/RSS TPW http://www.remss.com/measurements/atmospheric-water-vapor  (they report data for each month after the year end, 202312 means thru December, 2023) https://data.remss.com/vapor/monthly_1deg/tpw_v07r02_198801_202312.time_series.txt

6. Transportation fuel https://www.eia.gov/outlooks/ieo/pdf/transportation.pdf

7. Fuel properties https://en.wikipedia.org/wiki/Gasoline

8. World electricity generation https://yearbook.enerdata.net/electricity/world-electricity-production-statistics.html

9. Fuel sources for electricity generation https://www.eia.gov/outlooks/ieo/exec_summ.php

10. WV from coal combustion http://energyeducation.ca/encyclopedia/Water_vapour

11. Energy content of bituminous coal https://en.wikipedia.org/wiki/Energy_density

12. Global water withdrawal http://www.fao.org/nr/water/aquastat/water_use/index.stm

13. Irrigated agricultural area http://www.fao.org/nr/water/aquastat/infographics/Irrigation_eng.pdf

14. Annual fresh water withdrawal http://www.fao.org/nr/water/aquastat/didyouknow/index2.stm

15. 70% of withdrawal is for agriculture http://www.fao.org/nr/water/aquastat/infographics/Withdrawal_eng.pdf

16. Surface irrigation https://water.usgs.gov/edu/irfurrow.html

17. Frequency of furrow irrigation https://naldc.nal.usda.gov/download/54786/PDF

18. Pond evaporation rate http://www.nws.noaa.gov/oh/hdsc/Technical_papers/TP13.pdf

19. Climate change drivers http://globalclimatedrivers2.blogspot.com

20. Surface irrigation 86%: http://www.fao.org/3/I9253EN/i9253en.pdf

21. Ocean surface temperature animation https://www.youtube.com/watch?v=1ir1w3OrR4U

22. Modtran: http://forecast.uchicago.edu/Projects/modtran.orig.html

23. Modtran comparison with measured: http://climatemodels.uchicago.edu/modtran/modtran.doc.html

24. Atmospheric absorption of water vapor is logarithmic: https://wattsupwiththat.com/2016/07/25/precipitable-water/#comment-2264644

25. Bolton equation for water saturation p T https://glossary.ametsoc.org/wiki/Clausius-clapeyron_equation

26. Ice and mixed phase clouds: http://www.cas.manchester.ac.uk/resactivities/cloudphysics/background/ice/

27. Wexler, vapor pressure of water: https://www.ncbi.nlm.nih.gov/pmc/articles/PMC5312760/

28. Wexler, thermodynamic calculations for the vapor pressure of ice: https://nvlpubs.nist.gov/nistpubs/jres/81A/jresv81An1p5_A1b.pdf

29. Ocean temperatures: https://rwu.pressbooks.pub/webboceanography/chapter/6-2-temperature/

30. HadCRUT4 data: http://www.metoffice.gov.uk/hadobs/hadcrut4/data/current/time_series/HadCRUT.4.6.0.0.monthly_ns_avg.txt

31. HadCRUT5 data: https://www.metoffice.gov.uk/hadobs/hadcrut5/data/current/download.html

32. Theory of Redirected energy: https://energyredirect3.blogspot.com

33. Water vapor generated by humanity is 90% from irrigation: https://agupubs.onlinelibrary.wiley.com/doi/full/10.1029/2001WR000355

34. Ground level WV 5 g/kg ≈ 8000 ppmv in 1992: https://www.eso.org/gen-fac/pubs/astclim/espas/pwv/mockler.html  Fig 1

35. Ground level WV 8,000 ppm WV vs altitude Spectralcalc: https://www.spectralcalc.com/atmosphere_browser/modify_atmosphere.php

36. Water use: https://www.fao.org/aquastat/en/overview/methodology/water-use

37. Pearson coefficient calculator: https://www.socscistatistics.com/tests/pearson/default2.aspx

38. p-value calculator: https://www.danielsoper.com/Statcalc/calculator.aspx?id=44

39. Ave global WV: https://www.labxchange.org/library/items/lb:LabXchange:328c385b:html:1

40. Wallace and Hobbs: http://www.gnss-x.ac.cn/docs/Atmospheric%20Science%20An%20Introductory%20Survey%20(John%20M.%20Wallace,%20Peter%20V.%20Hobbs)%20(z-lib.org).pdf 

41. Ice core measurements of CO2 at Lawdome Antarctica: https://www.ncei.noaa.gov/pub/data/paleo/icecore/antarctica/law/law2006-co2-noaa.txt 

42. UAH 6.1 temperatures: https://www.nsstc.uah.edu/data/msu/v6.1/tlt/uahncdc_lt_6.1.txt